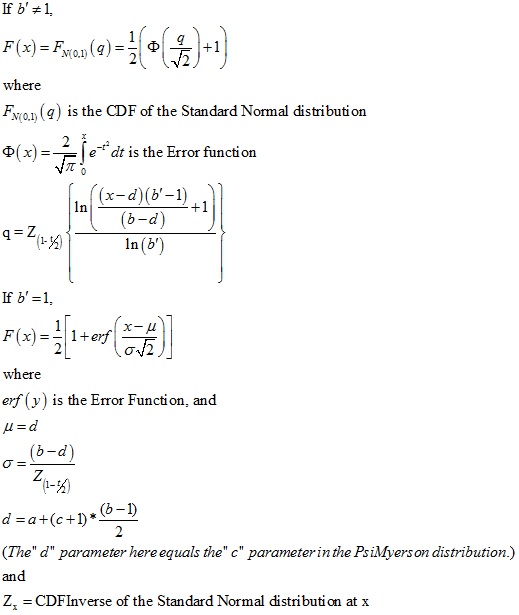
PsiNormalSkew(a,b,c,...)
PsiNormalSkew (a,b,c) is a generalized Normal distribution with lower bound a, upper bound b, and skew value c. Lower and upper bound values describe +/- 3rd standard deviation. The skew value c can take on values between (but not including) -1 and 1. While the Normal distribution is symmetric, the Normal Skew distribution is skewed either to the left with a positive skew parameter or to the right with a negative skew parameter.
Both the Myerson distribution (described above) and the PsiNormalSkew distribution have recently emerged in practice. Both distributions are generalizations of the Normal distribution but rather than using the mean and standard deviation as arguments, these distributions are calculated using an upper and lower bound along with either likely and tail arguments (such as with the Myerson distribution) or a skew argument (such as with the NormalSkew distribution). When the skew argument is equidistant from the upper and lower bounds, the NormalSkew distribution equals the Myerson distribution.
In the PsiNormalSkew distribution, the lower and upper bounds are exactly the same as in the Myerson distribution. The tail argument is missing in the Normal Skew distribution as it remains at the constant value of 0.002699796146511.